外分を含む平面ベクトル(その1)【たすきがけで比を統一せよ!!】

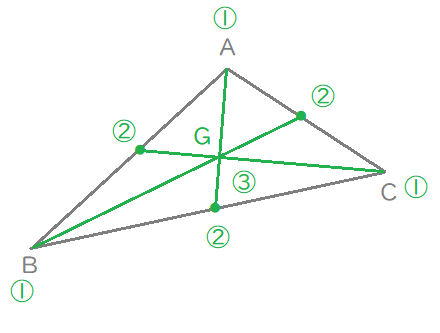
また三角形\(\mathrm{ABC}\)の重心を\(\mathrm{G}\)とし,\(\overrightarrow{\mathrm{GA}}=\overrightarrow{a}, \ \overrightarrow{\mathrm{GB}}=\overrightarrow{b}, \ \overrightarrow{\mathrm{GC}}=\overrightarrow{c}\)とする.
(1)
ⅰ) \(\overrightarrow{\mathrm{GD}}\)を\(\overrightarrow{a}, \ \overrightarrow{b}\)を用いて表せ.ここで,\(\mathrm{CF:FD}=t:\left( 1-t\right)\)とおく.
ⅱ)\(\mathrm{G}\)を重心とするとき,\(\overrightarrow{c}\)を\(\overrightarrow{a}, \ \overrightarrow{b}\)を用いて表せ.
ⅲ)\(\overrightarrow{\mathrm{GF}}\)を\(\overrightarrow{a}, \ \overrightarrow{b}, \ t\)を用いて表せ.
ⅳ)同様に\(\mathrm{BF:FE}=s:\left( 1-s\right)\)とおくとき,\(\overrightarrow{\mathrm{GF}}\)を\(\overrightarrow{a}, \ \overrightarrow{b}, \ s\)を用いて表せ.
ⅴ)\(s, \ t\)の値を求めよ。
(2) さらに,\(\vert \overrightarrow{b} \vert=1\)かつ,線分\(\mathrm{GA}\)と線分\(\mathrm{GF}\)の長さについて\(\mathrm{GA:GF}=3:2\)のとき,\(\overrightarrow{a},\overrightarrow{b}\)の内積\(\overrightarrow{a}\cdot \overrightarrow{b}\)を求めよ.
「外分」でも「内分」と同じように。

Lukia
「外分比」は一見難しそうですが、ためしに「内分比」と同じように各頂点に比をおいてみたところ、特に問題は生じませんでした。
ちょっとコツはあるので、そこを説明します。

Lukia

「辺\(\mathrm{AB}\)を\(4:1\)に外分する点\(\mathrm{D}\)」の打ち方と比の置き方です。
頂点\(\mathrm{B}\)の上に比の4(図では〇で囲んでいます)を書き、頂点\(\mathrm{A}\)には\(\color{#0004fc}{-1}\)を書きます。
本来、外分点\(\mathrm{D}\)は、線分\(\mathrm{AB}\)の延長線上にあり、3点は\(\mathrm{A, \ B, \ D}\)と並びます。
しかし、\(\color{#0004fc}{-}\)(マイナス)をつけて表すことで、「内分比」と同じように扱うことができるのです。
ゆえに点\(\mathrm{D}\)の比は、両端点の和である\(3\)となります。
同様に辺\(\mathrm{AC}\)を\(3:1\)に外分する点\(\mathrm{E}\)についても、頂点\(\mathrm{A}\)の上に書く比を\(\color{#0004fc}{-1}\)とおくことで、
内分点のように扱うことができます。
準備
$$\begin{align}\overrightarrow{\mathrm{GA}}+\overrightarrow{\mathrm{GB}}+\overrightarrow{\mathrm{GC}}=&\overrightarrow{0}\quad より, \\\\ \overrightarrow{a}+\overrightarrow{b}+\overrightarrow{c}=&\overrightarrow{0}\quad \cdots \ *\end{align}$$
![]()
(1)を解く。
ⅰ)
$$\begin{align}\overrightarrow{\mathrm{GD}}=&\overrightarrow{\mathrm{AD}}-\overrightarrow{\mathrm{AG}} \\\\ =&\displaystyle\frac{4}{3}\overrightarrow{\mathrm{AB}}+\overrightarrow{a} \\\\ =&\displaystyle\frac{1}{3}\left( 4\overrightarrow{b}-4\overrightarrow{a}+3\overrightarrow{a}\right)\\\\ =&-\displaystyle\frac{1}{3}\overrightarrow{a}+\displaystyle\frac{4}{3}\overrightarrow{b} \end{align}$$
ⅱ)
*より
$$\begin{align}\overrightarrow{c}=&-\overrightarrow{a}-\overrightarrow{b}\end{align}$$
ⅲ)
$$\begin{align}\overrightarrow{\mathrm{GF}}=&t\overrightarrow{\mathrm{GD}}+\left( 1-t\right)\overrightarrow{\mathrm{GC}} \\\\ =&\displaystyle\frac{1}{3}\lbrace -t\overrightarrow{a}+4t\overrightarrow{b}-3\left( 1-t\right)\overrightarrow{a}-3\left( 1-t\right)\overrightarrow{b}\rbrace \\\\ =&\displaystyle\frac{1}{3}\lbrace \left( 2t-3\right)\overrightarrow{a}+\left( 7t-3\right)\overrightarrow{b}\rbrace\\\\ =&\left( \displaystyle\frac{2}{3}t-1\right) \overrightarrow{a}+\left( \displaystyle\frac{7}{3}t-1\right)\overrightarrow{b}\quad \cdots \ ① \end{align}$$
ⅳ)
$$\begin{align}\overrightarrow{\mathrm{GF}}=&\left( 1-s\right)\overrightarrow{\mathrm{GB}}+s\overrightarrow{\mathrm{GE}} \\\\ =&\left( 1-s\right)\overrightarrow{b}+\displaystyle\frac{s}{2}\left( -\overrightarrow{a}+3\overrightarrow{c}\right) \\\\ =&\left( 1-s\right)\overrightarrow{b}+\displaystyle\frac{s}{2}\left( -4\overrightarrow{a}-3\overrightarrow{b}\right)\\\\ =&-2s\overrightarrow{a}+\left( 1-\displaystyle\frac{5}{2}s\right)\overrightarrow{b}\quad \cdots \ ②\end{align}$$
ⅴ)
①と②より
$$\begin{align}\displaystyle\frac{2}{3}t-1=&-2s \\\\ \displaystyle\frac{7}{3}t-1=&1-\displaystyle\frac{5}{2}s\\\\ \\\\連立方程式を解いて\\\\ &s=\displaystyle\frac{1}{3},\quad t=\displaystyle\frac{1}{2} \end{align}$$
(2)を解く。
条件より
$$\begin{align}\vert \overrightarrow{a} \vert:\vert \overrightarrow{\mathrm{GF}} \vert=& 3:2\\\\ 2\vert \overrightarrow{a} \vert=&3\vert \overrightarrow{\mathrm{GF}} \vert \\\\ 両辺を2乗して& \\\\ 4\vert \overrightarrow{a} \vert^2=&9\vert \overrightarrow{\mathrm{GF}} \vert^2 \\\\ =&9\vert -\displaystyle\frac{2}{3}\overrightarrow{a}+\displaystyle\frac{1}{6}\overrightarrow{b} \vert^2\\\\ =&4\vert \overrightarrow{a} \vert^2-2\overrightarrow{a}\cdot \overrightarrow{b}+\displaystyle\frac{1}{4}\\\\ \\\\ 2\overrightarrow{a}\cdot \overrightarrow{b}=&\displaystyle\frac{1}{4}\\\\ \overrightarrow{a}\cdot \overrightarrow{b}=&\displaystyle\frac{1}{8}\end{align}$$
こたえ
| (1) | ⅰ | $$\overrightarrow{\mathrm{GD}}=-\displaystyle\frac{1}{3}\overrightarrow{a}+\displaystyle\frac{4}{3}\overrightarrow{b}$$ | |
| ⅱ | $$\overrightarrow{c}=-\overrightarrow{a}-\overrightarrow{b}$$ | ||
| ⅲ | $$\overrightarrow{\mathrm{GF}}=\left( \displaystyle\frac{2}{3}t-1\right) \overrightarrow{a}+\left( \displaystyle\frac{7}{3}t-1\right)\overrightarrow{b}$$ | ||
| ⅳ | $$\overrightarrow{\mathrm{GF}}=-2s\overrightarrow{a}+\left( 1-\displaystyle\frac{5}{2}s\right)\overrightarrow{b}$$ | ||
| ⅴ | $$s=\displaystyle\frac{1}{3}$$ | $$t=\displaystyle\frac{1}{2}$$ | |
| (2) | $$\overrightarrow{a}\cdot \overrightarrow{b}=\displaystyle\frac{1}{8}$$ | ||
 以下の記事一覧に他のボリュームのブログカードを載せています。
以下の記事一覧に他のボリュームのブログカードを載せています。途中のボリュームからお読みになった方はこちらからどうぞ。










ディスカッション
コメント一覧
まだ、コメントがありません